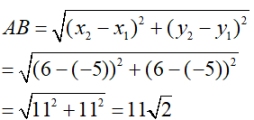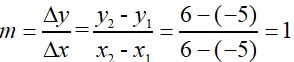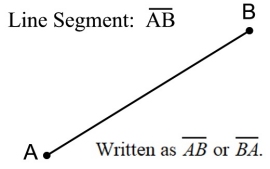
GeometrySmallLogo

Directed Line Segments - Introduction
MathBitsNotebook.com

Topical Outline | Geometry Outline | MathBits' Teacher Resources

Terms of Use   Contact Person: Donna Roberts
divider
Throughout this Geometry course, we have been working with line segments, such as .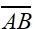
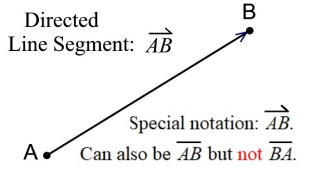
We have seen that this segment can be referred to as eithe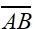r or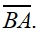

In this lesson, we are going to be examining a "directed" line segment,
where "direction" in relation to the segment will be important.

def
A directed segment is a segment that has distance (length) and direction. It is important to understand that a directed segment has a "starting point" (called the "initial point"), and an "ending point" (called the terminal point).

Directed line segments are often used to represent vectors.

Notation: Due to their association with vectors, you may see directed line segments with the
"modified arrow"   notation
which is commonly used with vectors.

In coordinate geometry, however, a directed line segment is likely to be referenced simply by a statement of the endpoint coordinates of the segment, thus avoiding any symbolic notation.
Once the directed line segment has been identified in a problem, you may then see references such as to refer to the computations on the segment portion.

divider

When working in the coordinate plane, ORDER MATTERS!
Label the initial point, A, (x1, y1), and label the terminal point, B, (x2, y2).
Order is important in controlling the "direction" on the coordinate plane,
as to whether a direction is positive (increasing) or negative (decreasing) in its movement.

 

Directed line segments and distance (length) in the Coordinate Plane:

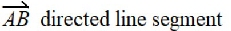A directed line segment goes from
point A(-5,5) to point B(6,6).

The length of the segment (distance)
from point A to point B
can be determined
by the Pythagorean Theorem,
(AB)2 = 112 + 112

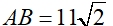
or by the Distance Formula,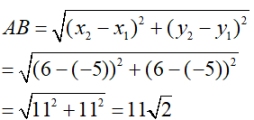


divider

Directed line segments and direction (slope) in the Coordinate Plane:


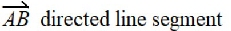A directed line segment goes from
point A(-5,5) to point B(6,6).

The direction of the segment
from point A to point B
can be represented by
the slope of the segment.

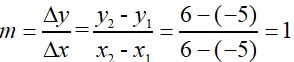
positive slope, rise 1, run 1
(from lower left to upper right)

Remember, when labeling the (x,y) coordintes, label the initial point as (x1,y1)
and the terminal point as (x2,y2).

If this ordering is reversed, such that Δx = x1 - x2,
the horizontal run would be -11, which is not true in this example.

divider

See the next lessons:
Directed Line Segments: Partitions and Ratios
Partition Directed Line Segments: Methods


divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation and is not considered "fair use" for educators. Please read the "Terms of Use".