Construct a Circle Inscribed in a Triangle MathBitsNotebook.com Topical Outline | Geometry Outline | MathBits' Teacher Resources Terms of Use   Contact Person: Donna Roberts

Remember -- use your compass and straightedge only!

Intuitively, the word "inscribed", in geometry, refers to one shape "fitting snugly"
inside of another geometric shape.

An inscribed circle in a triangle is the largest circle that can be drawn within the triangle, that is tangent to (just touches in one point) all three sides of the triangle. You may see an "inscribed circle" referred to as an incircle.

inscribed = inside

See the discussion on "incenters" at Incenter - Concurrent ∠ Bisectors.

When we circumscribed a circle about a triangle, we easily determined the radius of the circle by measuring the distance from the center to a vertex. When constructing an inscribed circle in a triangle, we can try to "eyeball" the radius of our circle, but we have no actual length to measure. We need an actual length, since "eyeballing" is not sufficient in a formal construction.

To determine the length of the radius, we will construct a perpendicular to one side of the triangle from the center point to get the actual length. We will be applying the theorem below, where the "tangent line" is the side of the triangle:

Theorem: The radius of a circle drawn to the point of tangency (of a tangent line)
is perpendicular to the tangent.

Given: an acute scalene triangle ΔABC Construct: Inscribe the circle in the triangle. Construct a circle inside the triangle that will be tangent to all three sides of the triangle.

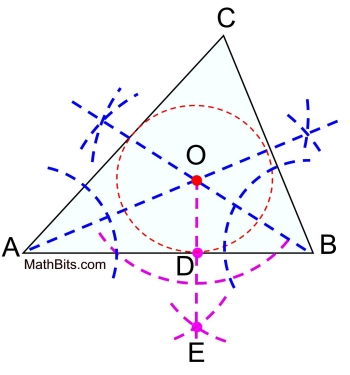
This construction will focus on the angle bisectors of the angles of the triangle. Remember the theorem: "If a point lies anywhere on an angle bisector, that point is equidistant from the two sides (rays) of the bisected angle." Where the angle bisectors intersect (say point O), will be used to establish that OD = OF = OG as the radii of a circle tangent to the sides of the triangle.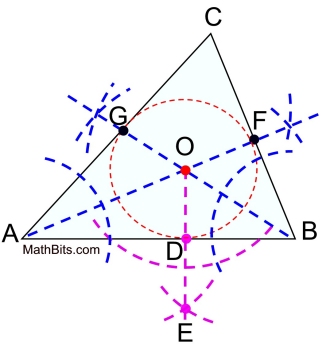

Be careful with this construction. Accuracy is very important. If you want your circle to touch all three sides of the triangle, you will need to be as accurate as possible with each step in this construction. Remember, the circle should just touch each of the sides.

Only two angle bisectors will be needed. We know OF = OD and OG = OD since any point on the angle bisector is equidistant from the sides (rays) of the angle. The substitution (or transitive) property gives us that OF = OG, and we have all three radii equidistant from the sides (rays) of the angles, OF = OG = OD. It is not necessary to have the third angle bisector, since we already know mathematically that OF = OG. Yes, you can construct the third angle bisector, if you wish, to verify the location of point O.

Proof of Construction: Since point O lies on the bisector of ∠A, point O is equidistant from and , since any point on the bisector of an angle is equidistant from the sides of the angle. Since O also lies on the bisector of ∠B, point O is equidistant from both and .
Therefore, point O is equidistant from , and . The length of the perpendicular measures the distance from O to . A circle whose center is O, with a radius of OD, will be tangent to the sides of ΔABC (touching only in one point). This gives us circle O inscribed in ΔABC.