1. (52)5 = 510
Power raised to a power → multiply the exponents. |
2. ((-10)3)5 = (-10)15
Negative number base: Power raised to a power → multiply the exponents.
|
3. 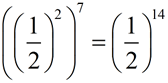
Fraction base: Power raised to a power → multiply the exponents.
|
4. 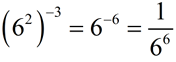
One of the exponents is negative. Still follow the rule and multiply the exponents. Be careful of the sign. In this case a positive number times a negative number gives a negative number. |
5. (a4)3 = a12
Variable base: Power raised to a power → multiply the exponents.
|
6. 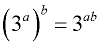
Numerical base with variable powers.
|
7. 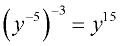
Power raised to a power → multiply the exponents.
Negative exponents at work, but not for long.
|
8. 
Result has a negative exponent. Do not leave the negative exponent as an answer.
|
9. (x2)a+2 = x2(a+2) = x2a+4
Power raised to a power → multiply the exponents.
The Distributive Property is also at work here.
|
10. Negative exponent with quotient: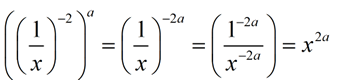 |